home

introduction

cs

platform

vns

platform

drive

system

planning

construction

plan

Choice of platform type 

Determining the center of gravity of telescope and platform table

Graphical determination of the length of the platform

Graphical determination of the width of the platform and of the radius of the circle segment

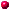Transformation of the circle segment into VNS segments

The platform type that is the easiest to understand is the circle segment (CS) platform with a tilted circle segment as northern bearing. This platform type has several disadvantages and does not allow in particular for a true three-point support. 

Somewhat more complex are the VNS platforms with vertical north segments. The offer a number of advantages, such as an easier construction of the roller bearings, a more direct weight transmission, a true three-point support, and a higher load capacity. 

A further option addresses the way the Dobsonian telescope is mounted on the platform. 

You may put your Dob together with its ground board onto the platform. In this case it is useful to add fixtures on the platform table that keep the feet of the Dob's ground board in place. 

This options is particularly useful for smaller Dobsonians, for which the additional increase of eyepiece height by the ground board does not matter. Furthermore, the ground board remains an integral part of the Dob, which can be used with or without platform without the need to mount or dismount anything. 

For larger Dobsonian telescopes, the eyepiece height is an issue. Therefore it is useful to save the height of the ground board and let the platform table take over its role. In this case the guiding rollers that hold the rocker box (for rocker boxes with round cut-outs at the bottom) or the central bolt (for rocker boxes with conventional azimuth bearings) can be attached to the platform table together with the Teflon pads. In the latter case (rocker boxes with traditional central bolt) you may not cut out the center of the platform table. Due to its easier mounting and dismounting, a rocker box design with cut-out bottom as in the picture to the right is of advantage.

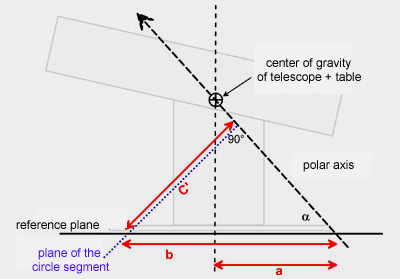
In the cartoon to the right, the most important measures of the platform and the telescope are summarized. In the following we will learn step by step, how to calculate these measures. As mentioned above, we will take the upper side of the platform table as reference plane. The angle alpha corresponds to the geographic latitude, the angle beta is 90° - alpha. 

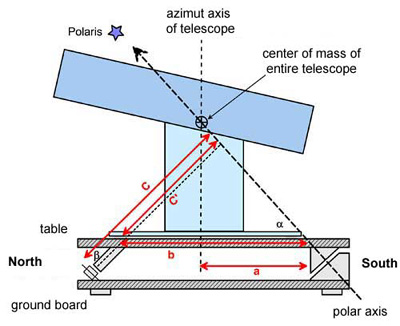
As a first step, we draw the reference plane and the polar axis in an angle alpha corresponding to your latitude. To support the telescope in its center of gravity, this center of gravity needs to be on the polar axis (= axis of rotation). This determines the distance a between the intersection of the azimuth axis and the polar axis with the reference plane.

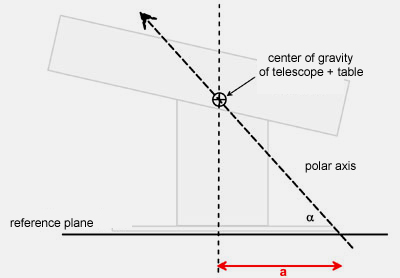
In a next step, we will switch into the reference plane. We draw a circle around the intersection point with the azimuth axis corresponding to the size of the azimuth bearing surface (e.g. the ebony star ring under the rocker box), such that the Teflon pads (or alternatively the feet of the ground board, if this will be used on the platform) are just within this circumference. One of the Teflon pads points toward south, while the positions of the other two will define the intersection line of the future circle segment with the reference plane. From the drawing, you can further determine the distance b - a.

Now, we switch back into the side perspective. The distance b is now defined. Next, we will draw the plane of the future circle segment, which is perpendicular to the polar axis. The distance between polar axis and intersection with the reference plane is c'.

In the next step we switch into the plane of the circle segment. We draw the intersection of the polar axis (= center of the future circle segment) and in a distance c' the intersection of the circle segment plane with the plane of reference.

We can now place the two northern Teflon pads along this intersection line. The future circle segment (and thus the width of the platform) should be somewhat wider than the distance between the Teflon pads (in practice about 10 cm on each side, half of the length of the tracking surfaces). For 1 1/2 hours of tracking, the length of the tracking surface (corresponding approximately to d) is

(1.5h/24h) x 2 c pi

With a pair of compasses we can now draw the circle segment between the two green arrows and determine its radius c as well as the width of the platform e.

For Dobs with relatively small ground board and/or high center of gravity, this method would lead to a narrow, long platform. In this case, it may be useful to make the circle segment somewhat wider. The Teflon pads will then be no longer in the middle of the tracking surfaces, but are shifted toward their inner ends. 

For a VNS platform, we still need to make the transformation from the circle segment to the elliptical VNS segments. This will be achieved in two steps. 

As explained already here, the usual circular segment for the northern bearing is only a special case of a continuum of possible shapes, which all are sections of a cone. This cone is defined by the polar axis and an angle, which is slightly larger than the geographical latitude (otherwise the segment would not be below the horizontal table). For a circular segment, the plane of the section is perpendicular to the polar axis. In our alternative approach, we can put the plane of the section perpendicular to the platform table. What we get is an ellipse or an elliptical or hyperbolic segment (see conic sections on Wikipedia).

The advantage of vertical northern segments (VNS) is the more direct transmission of the weight of the Dob to the ground plate and the simpler design of the roller bearings and the motor drive. 

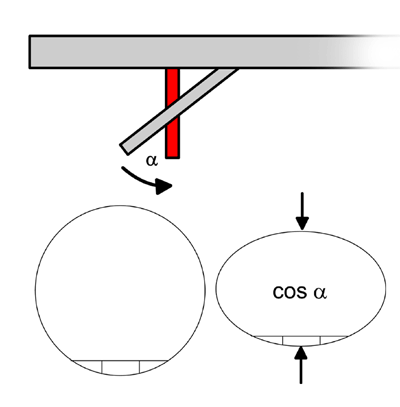
The shape of the VNS segments can be calculated and drawn to scale. This can be done analytically, but this is complicated. It is much more simple to start from the inclined circular segment. The shape of the vertical elliptical segment is obtained by a simple projection of the inclined circle segment into a vertical plane, followed by dividing of the segment into two parts and a slight rotation of each of the parts around a vertical axis. 

By the projection into the vertical plane, the circle is compressed by a factor of cos alpha (where alpha is again the geographical latitude). The circle segment turns to an elliptical segment. This procedure is best done using some simple graphic software, from which you can print out the part of the segment that you need (even Powerpoint could do this). It is helpful to draw also the position of the reference plane (the horizontal line in the scheme) and the area needed for the tracking surfaces (marked by vertical lines).

In the next step, the elliptical segment are split into two segments, that are slightly rotated by an angle beta around a vertical axis such that they are perpendicular to the line connecting them with the southern bearing. This will align the segments with the movement of the platform table and decreases the amount of lateral movement of the segment on the rollers during operation of the platform. To account for this, the segments need to be stretched by a factor 1/ cos beta.

The segment (only that part that is needed) can then be printed on a sheet of paper and serve as a jig for cutting out the segments. 

In contrast to the circle segment, the points on the tracking surface of a VNS segment do not maintain precisely the same distance to the southern pivot point. With some brainstorming, one can deduce that the required tracking speed is no longer constant but becomes a function of the position of the tracking surface. One can, however, determine the deviation from an average tracking speed, which is less than +/- 1%.